

Using the equation above to calculate the 5 th term: EX: a 5 = a 1 + f × (n-1) It is clear in the sequence above that the common difference f, is 2. The general form of an arithmetic sequence can be written as: This difference can either be positive or negative, and dependent on the sign will result in terms of the arithmetic sequence tending towards positive or negative infinity. Arithmetic SequenceĪn arithmetic sequence is a number sequence in which the difference between each successive term remains constant. Indexing involves writing a general formula that allows the determination of the n th term of a sequence as a function of n. In cases that have more complex patterns, indexing is usually the preferred notation. There are multiple ways to denote sequences, one of which involves simply listing the sequence in cases where the pattern of the sequence is easily discernible.
Infinite fraction converter series#
They are particularly useful as a basis for series (essentially describe an operation of adding infinite quantities to a starting quantity), which are generally used in differential equations and the area of mathematics referred to as analysis. Sequences are used to study functions, spaces, and other mathematical structures. A series is convergent if the sequence converges to some limit, while a sequence that does not converge is divergent. Sequences have many applications in various mathematical disciplines due to their properties of convergence. There are many different types of number sequences, three of the most common of which include arithmetic sequences, geometric sequences, and Fibonacci sequences. In a number sequence, the order of the sequence is important, and depending on the sequence, it is possible for the same terms to appear multiple times. The individual elements in a sequence is often referred to as term, and the number of terms in a sequence is called its length, which can be infinite. Accordingly, a number sequence is an ordered list of numbers that follow a particular pattern. In mathematics, a sequence is an ordered list of objects. Preferable reference for this tutorial is ' Dim a(25) ' I assume the accuracy is lower than 25 digit decimals Dim y(25), r(25) Dim p(25), q(25) Dim error Dim i As Integer, k As Integer Dim ratio As Double, prevRatio As Double For k = 0 To 24 If k = 0 Then a(0) = Int(decNum) ' function Int in VB is equivalent to Floor r(0) = decNum - a(0) If r(0) 0 Then y(0) = 1 / r(0) p(0) = a(0) q(0) = 1 ratio = p(0) / q(0) error = Abs(decNum - ratio) Else a(k) = Int(y(k - 1)) ' function Int in VB is equivalent to Floor r(k) = y(k - 1) - a(k) y(k) = 1 / r(k) If k = 1 Then p(1) = a(1) * p(0) + 1 q(1) = a(1) * q(0) Else p(k) = a(k) * p(k - 1) + p(k - 2) q(k) = a(k) * q(k - 1) + q(k - 2) End If ratio = p(k) / q(k) error = Abs(ratio - prevRatio) End If If error
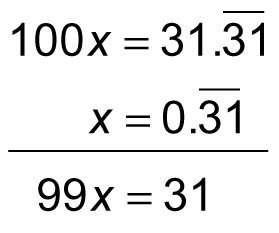
Infinite fraction converter code#
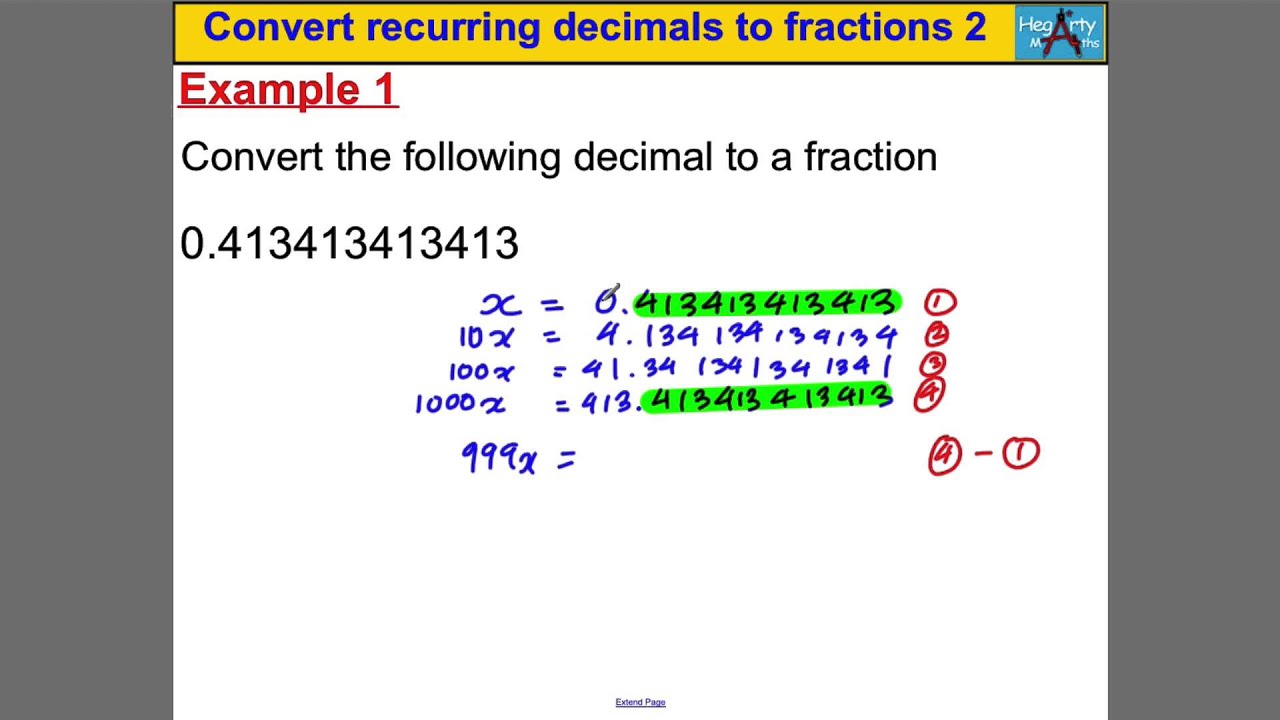
Visual Basic code for this conversion is given belowįunction Decimal2Fraction(ByVal decNum As Double, ByVal accuracy As Double, _ ByRef nominator, ByRef denominator, ByRef remainder) ' Input decNum always between zero to 1 , thus we stop the iteration and report the conversion of decimal We use difference equation with initial condition We start with making a continued fraction. We want to get the fraction approximation. The iteration is stop when the absolute error is very smallĪnd we report the result of one iteration before. Once we get the term of continued fraction, we can use the difference equation Then continued the iteration with iteration formula May not have the exact decimal number and we can use approximation of continued fraction using this simple algorithm:Īt the beginning, we compute term, error and reciprocal of remainder respectively as Of course, we can always say that 2.14567 = 214567/100000 without any approximation.


 0 kommentar(er)
0 kommentar(er)
